Some gamblers might he tempted to base staking plans on the theory that in any series of even-money events there must come a time sooner or later when the outcomes reach equipartition.

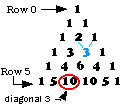
A glance at Pascal’s Triangle will answer the following question. When tossing a coin, what is the probability that after n events, the number of heads will equal the number of tails? Clearly this can happen only with an even number of events. Those who believe the ‘law of averages’ fallacy maintain that the probability of equipartition, as it is called, increases with the number of events. Pascal’s Triangle proves the opposite. Line 4 shows that if we toss four times, there are 16 Possible outcomes, of which six contain two heads and two tails. The Probability of equipartition is 6/16. Line 6 shows that with six tosses, the probability of equipartition is 20/64. Line 10 shows that with ten tosses, equipartition is a 252/1024 chance. The probabilities are becoming smaller as the tosses increase. The formula to discover the probability of equipartition in n events is to divide the number of combinations which give equipartition by the number of possible outcomes.

Example :-

What is the probability, when tossing six dice, of throwing each number once i.e. achieving equipartition?

The total number of ways equipartition can occur is 6! The first die can clearly be any of the six numbers. the second die one of the five remaining, and so on, giving a total number of ways of 6 x 5 x 4 x 3 x 2 x 1 = 720.

The number of possible outcomes is power(6,6) since there are six ways each of the six dice can fall. Therefore this is equal to 46656.

So the probability of equipartition is:-

720/46656 = 0.0154 or 1.5432%

Most people would be surprised to discover that if you threw six dice, on about 98.5% of occasions, at least one number will appear more than once.

If there are seven children in a family, what is the probability that they were born on different days of the week?

This is a question of equipartition, the answer being =fact(7)/power(7,7). The answer is0.6120%.